Theorem 1stconst 5842

Description: The mapping of a restriction of the 1st function to a constant function. (Contributed by NM, 14-Dec-2008.)

Assertion

Ref	Expression
1stconst	|- ( B e. V -> ( 1st |` ( A X. { B } ) ) : ( A X. { B } ) -1-1-onto-> A )

Proof of Theorem 1stconst

Dummy variables x y are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		snmg 3486	. . 3 |- ( B e. V -> E. x x e. { B } )
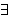
2		fo1stresm 5788	. . 3 |- ( E. x x e. { B } -> ( 1st |` ( A X. { B } ) ) : ( A X. { B } ) -onto-> A )
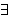
3	1, 2	syl 14	. 2 |- ( B e. V -> ( 1st |` ( A X. { B } ) ) : ( A X. { B } ) -onto-> A )
4		moeq 2716	. . . . . 6 |- E* x x = <. y , B >.
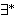
5	4	moani 1970	. . . . 5 |- E* x ( y e. A /\ x = <. y , B >. )
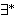
6		vex 2560	. . . . . . . 8 |- y e. _V
7	6	brres 4618	. . . . . . 7 |- ( x ( 1st |` ( A X. { B } ) ) y <-> ( x 1st y /\ x e. ( A X. { B } ) ) )
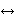
8		fo1st 5784	. . . . . . . . . . 11 |- 1st : _V -onto-> _V
9		fofn 5108	. . . . . . . . . . 11 |- ( 1st : _V -onto-> _V -> 1st Fn _V )
10	8, 9	ax-mp 7	. . . . . . . . . 10 |- 1st Fn _V
11		vex 2560	. . . . . . . . . 10 |- x e. _V
12		fnbrfvb 5214	. . . . . . . . . 10 |- ( ( 1st Fn _V /\ x e. _V ) -> ( ( 1st ` x ) = y <-> x 1st y ) )
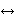
13	10, 11, 12	mp2an 402	. . . . . . . . 9 |- ( ( 1st ` x ) = y <-> x 1st y )
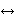
14	13	anbi1i 431	. . . . . . . 8 |- ( ( ( 1st ` x ) = y /\ x e. ( A X. { B } ) ) <-> ( x 1st y /\ x e. ( A X. { B } ) ) )
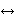15		elxp7 5797	. . . . . . . . . . 11 |- ( x e. ( A X. { B } ) <-> ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) e. A /\ ( 2nd ` x ) e. { B } ) ) )
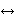
16		eleq1 2100	. . . . . . . . . . . . . . 15 |- ( ( 1st ` x ) = y -> ( ( 1st ` x ) e. A <-> y e. A ) )
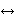
17	16	biimpa 280	. . . . . . . . . . . . . 14 |- ( ( ( 1st ` x ) = y /\ ( 1st ` x ) e. A ) -> y e. A )
18	17	adantrr 448	. . . . . . . . . . . . 13 |- ( ( ( 1st ` x ) = y /\ ( ( 1st ` x ) e. A /\ ( 2nd ` x ) e. { B } ) ) -> y e. A )
19	18	adantrl 447	. . . . . . . . . . . 12 |- ( ( ( 1st ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) e. A /\ ( 2nd ` x ) e. { B } ) ) ) -> y e. A )
20		elsni 3393	. . . . . . . . . . . . . 14 |- ( ( 2nd ` x ) e. { B } -> ( 2nd ` x ) = B )
21		eqopi 5798	. . . . . . . . . . . . . . 15 |- ( ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) = y /\ ( 2nd ` x ) = B ) ) -> x = <. y , B >. )
22	21	an12s 499	. . . . . . . . . . . . . 14 |- ( ( ( 1st ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( 2nd ` x ) = B ) ) -> x = <. y , B >. )
23	20, 22	sylanr2 385	. . . . . . . . . . . . 13 |- ( ( ( 1st ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( 2nd ` x ) e. { B } ) ) -> x = <. y , B >. )
24	23	adantrrl 455	. . . . . . . . . . . 12 |- ( ( ( 1st ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) e. A /\ ( 2nd ` x ) e. { B } ) ) ) -> x = <. y , B >. )
25	19, 24	jca 290	. . . . . . . . . . 11 |- ( ( ( 1st ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) e. A /\ ( 2nd ` x ) e. { B } ) ) ) -> ( y e. A /\ x = <. y , B >. ) )
26	15, 25	sylan2b 271	. . . . . . . . . 10 |- ( ( ( 1st ` x ) = y /\ x e. ( A X. { B } ) ) -> ( y e. A /\ x = <. y , B >. ) )
27	26	adantl 262	. . . . . . . . 9 |- ( ( B e. V /\ ( ( 1st ` x ) = y /\ x e. ( A X. { B } ) ) ) -> ( y e. A /\ x = <. y , B >. ) )
28		simprr 484	. . . . . . . . . . . 12 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> x = <. y , B >. )
29	28	fveq2d 5182	. . . . . . . . . . 11 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> ( 1st ` x ) = ( 1st ` <. y , B >. ) )
30		simprl 483	. . . . . . . . . . . 12 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> y e. A )
31		simpl 102	. . . . . . . . . . . 12 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> B e. V )
32		op1stg 5777	. . . . . . . . . . . 12 |- ( ( y e. A /\ B e. V ) -> ( 1st ` <. y , B >. ) = y )
33	30, 31, 32	syl2anc 391	. . . . . . . . . . 11 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> ( 1st ` <. y , B >. ) = y )
34	29, 33	eqtrd 2072	. . . . . . . . . 10 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> ( 1st ` x ) = y )
35		snidg 3400	. . . . . . . . . . . . 13 |- ( B e. V -> B e. { B } )
36	35	adantr 261	. . . . . . . . . . . 12 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> B e. { B } )
37		opelxpi 4376	. . . . . . . . . . . 12 |- ( ( y e. A /\ B e. { B } ) -> <. y , B >. e. ( A X. { B } ) )
38	30, 36, 37	syl2anc 391	. . . . . . . . . . 11 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> <. y , B >. e. ( A X. { B } ) )
39	28, 38	eqeltrd 2114	. . . . . . . . . 10 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> x e. ( A X. { B } ) )
40	34, 39	jca 290	. . . . . . . . 9 |- ( ( B e. V /\ ( y e. A /\ x = <. y , B >. ) ) -> ( ( 1st ` x ) = y /\ x e. ( A X. { B } ) ) )
41	27, 40	impbida 528	. . . . . . . 8 |- ( B e. V -> ( ( ( 1st ` x ) = y /\ x e. ( A X. { B } ) ) <-> ( y e. A /\ x = <. y , B >. ) ) )
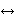42	14, 41	syl5bbr 183	. . . . . . 7 |- ( B e. V -> ( ( x 1st y /\ x e. ( A X. { B } ) ) <-> ( y e. A /\ x = <. y , B >. ) ) )
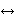
43	7, 42	syl5bb 181	. . . . . 6 |- ( B e. V -> ( x ( 1st |` ( A X. { B } ) ) y <-> ( y e. A /\ x = <. y , B >. ) ) )
44	43	mobidv 1936	. . . . 5 |- ( B e. V -> ( E* x x ( 1st |` ( A X. { B } ) ) y <-> E* x ( y e. A /\ x = <. y , B >. ) ) )
45	5, 44	mpbiri 157	. . . 4 |- ( B e. V -> E* x x ( 1st |` ( A X. { B } ) ) y )
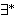46	45	alrimiv 1754	. . 3 |- ( B e. V -> A. y E* x x ( 1st |` ( A X. { B } ) ) y )
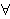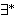
47		funcnv2 4959	. . 3 |- ( Fun `' ( 1st |` ( A X. { B } ) ) <-> A. y E* x x ( 1st |` ( A X. { B } ) ) y )
48	46, 47	sylibr 137	. 2 |- ( B e. V -> Fun `' ( 1st |` ( A X. { B } ) ) )
49		dff1o3 5132	. 2 |- ( ( 1st |` ( A X. { B } ) ) : ( A X. { B } ) -1-1-onto-> A <-> ( ( 1st |` ( A X. { B } ) ) : ( A X. { B } ) -onto-> A /\ Fun `' ( 1st |` ( A X. { B } ) ) ) )
50	3, 48, 49	sylanbrc 394	1 |- ( B e. V -> ( 1st |` ( A X. { B } ) ) : ( A X. { B } ) -1-1-onto-> A )

Syntax hints:   -> wi 4   /\ wa 97   <-> wb 98   A.wal 1241   = wceq 1243   E.wex 1381   e. wcel 1393   E*wmo 1901   _Vcvv 2557   {csn 3375   <.cop 3378   class class class wbr 3764   X. cxp 4343   `'ccnv 4344   |` cres 4347   Fun wfun 4896   Fn wfn 4897   -onto->wfo 4900   -1-1-onto->wf1o 4901   ` cfv 4902   1stc1st 5765   2ndc2nd 5766

This theorem was proved from axioms:  ax-1 5  ax-2 6  ax-mp 7  ax-ia1 99  ax-ia2 100  ax-ia3 101  ax-io 630  ax-5 1336  ax-7 1337  ax-gen 1338  ax-ie1 1382  ax-ie2 1383  ax-8 1395  ax-10 1396  ax-11 1397  ax-i12 1398  ax-bndl 1399  ax-4 1400  ax-13 1404  ax-14 1405  ax-17 1419  ax-i9 1423  ax-ial 1427  ax-i5r 1428  ax-ext 2022  ax-sep 3875  ax-pow 3927  ax-pr 3944  ax-un 4170

This theorem depends on definitions:  df-bi 110  df-3an 887  df-tru 1246  df-nf 1350  df-sb 1646  df-eu 1903  df-mo 1904  df-clab 2027  df-cleq 2033  df-clel 2036  df-nfc 2167  df-ral 2311  df-rex 2312  df-rab 2315  df-v 2559  df-sbc 2765  df-csb 2853  df-un 2922  df-in 2924  df-ss 2931  df-pw 3361  df-sn 3381  df-pr 3382  df-op 3384  df-uni 3581  df-iun 3659  df-br 3765  df-opab 3819  df-mpt 3820  df-id 4030  df-xp 4351  df-rel 4352  df-cnv 4353  df-co 4354  df-dm 4355  df-rn 4356  df-res 4357  df-ima 4358  df-iota 4867  df-fun 4904  df-fn 4905  df-f 4906  df-f1 4907  df-fo 4908  df-f1o 4909  df-fv 4910  df-1st 5767  df-2nd 5768

This theorem is referenced by: (None)